Quick Sort

Quick Sort :  Based on Divide and Conquer paradigm.

• One of the fastest in-memory sorting algorithms (if not the fastest)
• is a very efficient sorting algorithm
• designed by C.A.R.Hoare in 1960.
• Consists of two phases:
• Partition phase
• Sort phase

Steps…

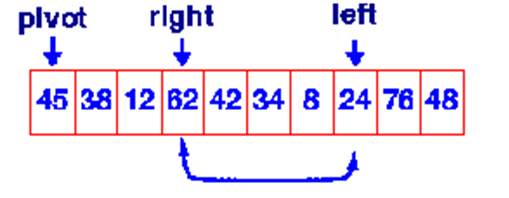
1. Divide: Pick a pivot element and rearrange the array so that

–  all elements to the left of the pivot are

smaller than the pivot.

–  all elements to the right of the pivot are larger

than the pivot.

2. Conquer: Recursively quicksort the left and right subarrays.

3:Combine: since subarrays are sorted in place, no work is needed to combine them, array is now sorted.

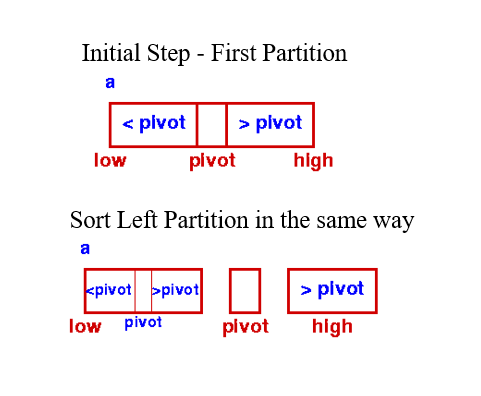
Quicksort – Partition phase

• Goals:
• Select pivot value
• Move everything less than pivot value to the left of it
• Move everything greater than pivot value to the right of it

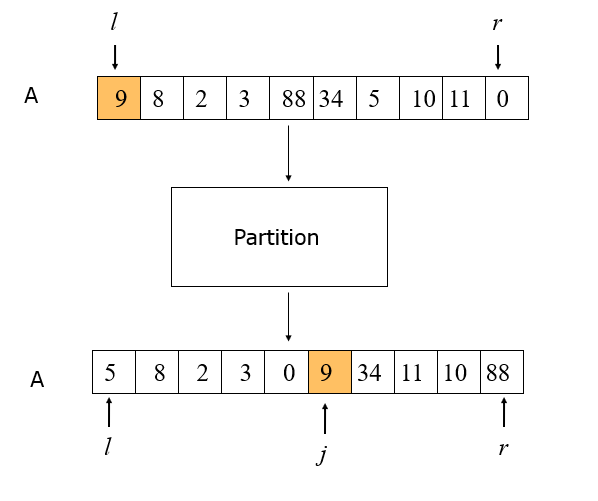
Algorithm:  Quick Sort

Procedure  QuickSort ( A, l, r )

if ( r > l ) then

j ¬ partition  ( A, l, r );

QuickSort ( A, l, j – 1 );

QuickSort ( A, j + 1 , r );

end of if.

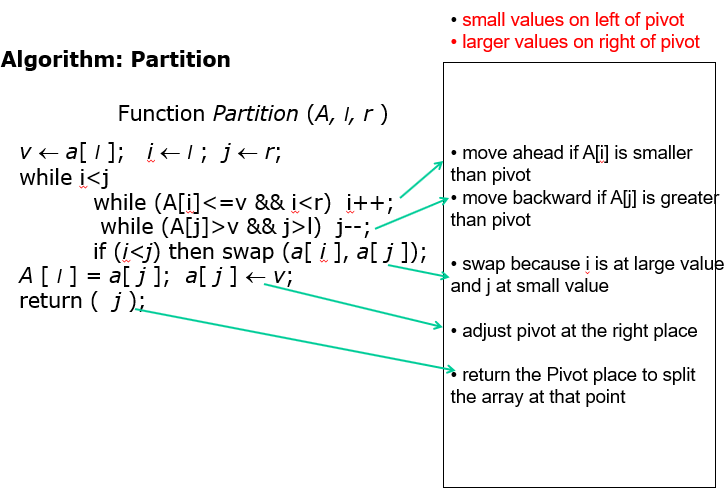
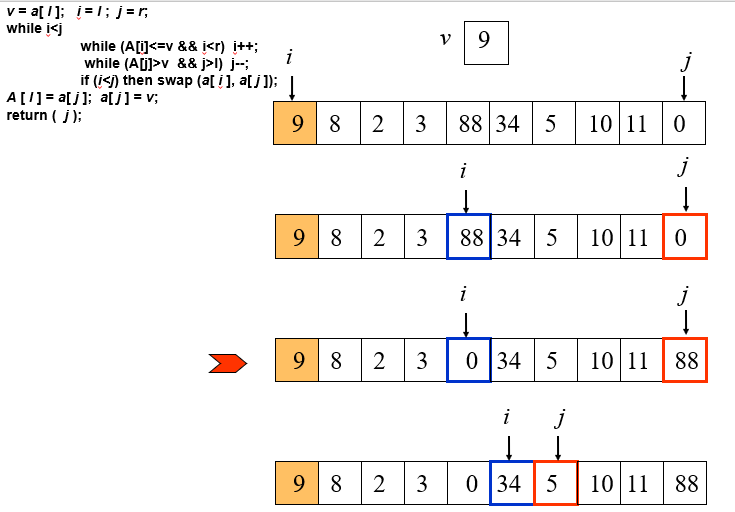
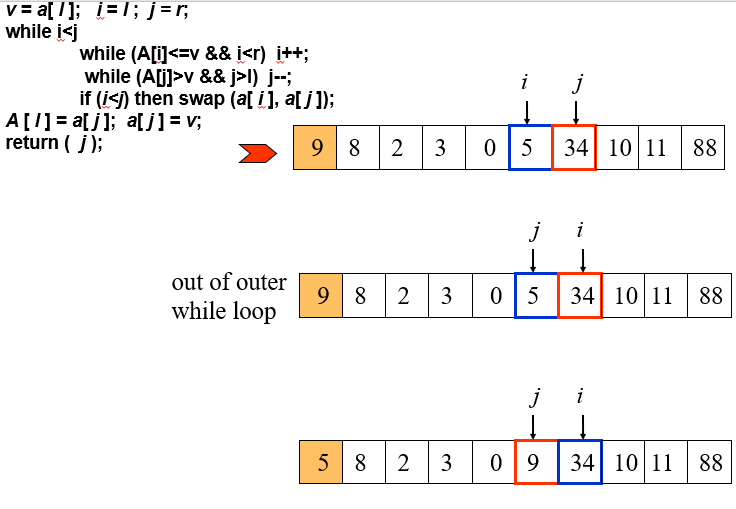
Function Partition (A, l, r )

v ¬ a[ l ];   i ¬ l ;  j ¬ r;

while i<j

while (A[i]<=v && i<r)  i++;

while (A[j]>v && j>l)  j–;

if (i<j) then swap (a[ i ], a[ j ]);

A [ l ] = a[ j ];  a[ j ] ¬ v;

return (  j );

There are various algorithms for partition.

Above Algorithm does not need an extra array.

Only 3 variables v,i,  and  j are used.

Time Complexity: Best case/average case

• Recurrence Relation

T(n)=2T(n/2) + n

Using Master Theorem applying case 2:

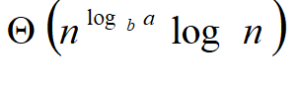
So time complexity is O(nlogn)

• Why is quick sort better than merge sort?
• Better memory consumption

 

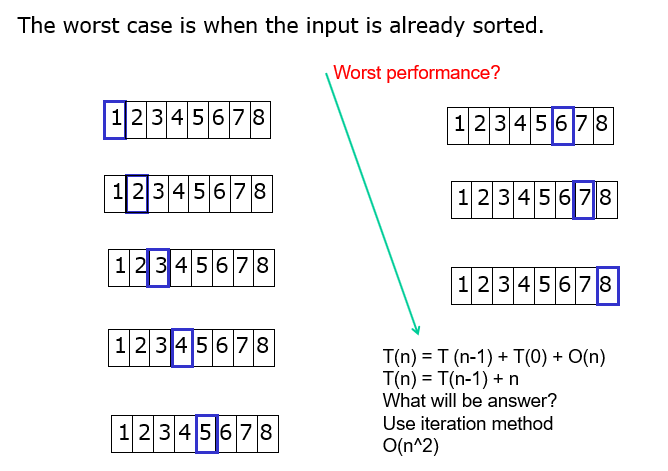
The worst case is when the input is already sorted.

Randomized Quick Sort

• Randomize the input data before giving it to Quick Sort. OR
• In the partition phase, rather than choosing first value to be the pivot value, choose a RANDOM value to be pivot value.
• This makes Quick Sort run time independent of input ordering
• So Worst case won’t happen for SORTED inputs but may only happen by worseness of random number generator.

C++ code  for quick sort using recursive function

#include <cstdlib>
#include <iostream>
#include<time.h>
#include<conio.h>
#define size 10

using namespace std;

void swap(int&a,int&b)
{
int tmp=a; a=b; b=tmp;
}

void quicksort(int a[],int left,int right)
{
int i=left,j=right,x=(left+right)/2,pivot=a[x];

while(1)
{
while(a[i]<pivot) i++;
if(i>x)break;
while(a[j]>pivot) j–;
if(j<x)break;
if(i<=j)
{
swap(a[i],a[j]);
i++;
j–;
}
}
if(j>left)
quicksort(a,left,j);

if(i<right)
quicksort(a,i,right);
}

int main()
{
srand(time(0));
int a[size],j=10;
for(int i=0;i<size;i++)
a[i]=rand()%100;

cout<<“Original Array: “;
for(int i=0;i<size;i++)
cout<<a[i]<<” “;
cout<<endl<<endl;

quicksort(a,0,size-1);
cout<<“Sorted Array: “;
for(int i=0;i<size;i++)
cout<<a[i]<<” “;
cout<<endl<<endl;
getch();
return EXIT_SUCCESS;
}

Related links 

Single link list                 Stack              AVL Trees             Binary search          Counting Sort

Doubly link list               Queue              Graphs                  Bubble Sort               Radix sort

Circular link list              Binary search tree       Hashing         Insertion Sort         Bucket Sort

Josephus Problem          Tree Traversal              Heaps                Quick Sort              Merge Sort

 

At Cui tutorial, courses, past papers and final year projects

#tutorial #cui #pastpaper #courses