Statistics and probability theory past/Previous question paper
– Statistics and probability theory project-Computer Science all subject past paper –Statistics and probability theory Problem with source code – Statistics and probability theory Course Statistics and probability theory Project idea -Statistics and probability theory MCQ Statistics and probability theory Interview Question –Computer Science all courses Statistics and probability theory concepts-University Past Paper – Statistics and probability theory –Question paper –old paper
Q1: a) What will be variance and standard deviation in each of the following cases if Var(X) =25
- 2X (2) X+/-2 (3) 3X+/-5
b) For a set of “n” values of X, it is known that

c) Prove that if A and B are any two events defined in a sample space S, then

e) A card is drawn from a well shuffled pack of 52 cards. Find the probability of (1) A non-face card (2)Neither a heart nor a red king (3) A non-ace
Q2: a) The following data is obtained from the survey. Calculate the average speed of cars by using suitable average method.
| Speed of the Car | 130 | 135 | 140 | 145 | 150 |
| No of Cars | 3 | 4 | 8 | 9 | 2 |
b) The following are the emergency room charges made to a sample of 25 patients at a city hospital. Use this data to prepare a frequency distribution and draw a histogram from the following figures. Also find out standard deviation and its coefficient by: (1) Taking actual mean (2) Taking Assumed Mean 199.50,186.00,173.20,186.00,214.10,125.50,143.50,190.40,152.00,165.70,154.70,145.30,154.60,190.30,135.40,167.70,203.40,186.70,155.30,195.90,168.90,166.70,178.60,150.20,212.40
c) If two dice are thrown, what are the various total number of dots that may turn up? What are the probabilities of each of them? What is the probability that the number of dots will total at least four?
d) A zip code contains 5 digits. How many different zip codes can be made with the digits 0-9 if no digit is used more than once and the first digit is not 0?
Statistics and probability theory Sessional I past paper in 2020
Question no 1: Marks: 10
(a) The following data is obtained from the survey. Calculate the average speed of cars by using suitable average method. [6]
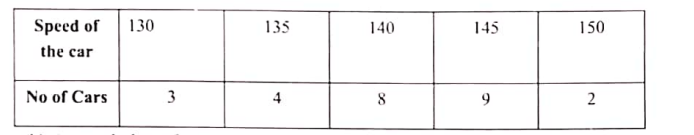
(b) A population of N-10 has the observations 7, 8, 10, 13, 14, 19, 20, 25, 26, and 28. Find its variance and standard Deviation. [4]
Question no 2:
Construct:
(a) Histogram (b) Frequency Polygon and (c) Cumulative Frequency curve from the following data:
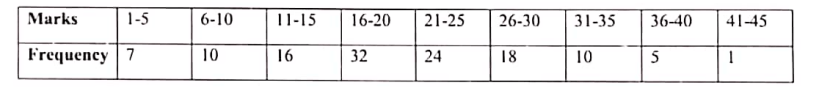
Find approximate value of the median from the ogive and check your answer by calculating.
Statistics and probability theory sessional II in 2020
Question no 1: Marks: 10
(a) Let A be the even that a family has children of both gender and B be the event that a family has at most one boy. If a family is known to have (i) three children, then show that A and B are independent events (ii) four children, then show that A and B are dependent events.
(b) One integer is chosen at random from the numbers 1, 2, 3.50. What is the probability that the chosen number is divisible by 6 or by 8
Question no 2:
(a) Two standard dice with 6 sides are thrown and the faces are recorded. Given that the sum of the two faces equal to 10, what is the probability that the first throw equals to 5? (4) (b) If one card is selected at random from a deck of 52 playing cards; what is the probability that the card is a club or a face card or both?
Marks: 10
(c) A box contains 15 items, 4 of which are defective and 11 are good. Two items are selected. What is the probability that the first is good and the second defective? (3)
(3)
Marks: 10
Question no 3:
(a) A die is cast twice and a coin is tossed twice. What is the probability that the die will tum a 6 each time and the coin will tum a tail every time?
(b) In a group of 20 adults, 4 out of the 7 women and 2 out of 13 men wear glasses. What the probability that a person chosen at random from the group is a woman or someone who wear glasses? (4)
(c) Two events A and B are such that P(A)-1/4, P(A/B)-1/2 and P(B/A)-2/3 (i) Are A and B independents events? (ii) Are A and B mutually exclusive events?
(iii) Find P(An B) and P(B).
Statistics and probability theory Final paper 4 in 2020
Question no 1:
L What is the shape of binomial distribution?
Fast PDE Sead
Π. Let “X” be a continuous random variable. What is the probability that “X” assumes a
single value, such as a? Explain the difference between y and 9. For the standard normal distribution, find the area within one and half standard deviation of the mean-that is the area between p-1.50 and +1.50.
IV. Find the probabilities for the standard normal distribution (a) P(-1.56 SzS 2.31).(b) P(Z >-.75)
Question no 2:
Marks: 8
I. Briefly explain the conditions that must hold true to use the t distribution to make a test of hypothesis about the population mean?
II. Write down the interpretation of Coefficient of determination r² when r² = 1 and r² = 0.
What type of error would you make if the null hypothesis is actually false and you fail to reject it? Why is the random error term included in a regression model?
IV. What are the signs in H. and H, and tails of a test of hypothesis? Show these outcomes by writing a table.
Question no 3:
(a) Determine the probability that the income tax authorities will catch 3 income tax returns with illegitimate deductions, if it randomly selects returns from among 20 income tax returns of which 8 contain illegitimate deductions. (3)
(b) The first print of a new book consisting of 750 pages has on an average 1 error in five pages. Find the number of pages which have 0, 1, 2, 3 errors per page in the whole book. (3)
(c) A manufacturing of television set known that on an average 5% of their product is defective. They sell television sets in consignment of 100 and guarantees that not more than 2 set will be defective. What is the probability that the TV set will fail to meet the guaranteed quality.
(4)
Question no 4:
(a)A diabetic is interested to determining how the amount of serobic exercise impacts his blood sugar. When his blood sugar reaches 170 mg/dl., he goes out for a run at a pace of 10 minutes per mile. On different days, he runs different distance and measures his blood sugar after completing his run. Note: the preferred blood sugar level is in the range of 80 10 120 mg/dl. Levels that are too low or too high are extremely dangerous. The data generated are given in the following table.
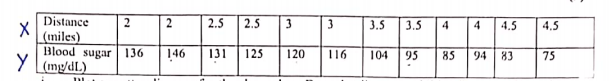
Plot a scatter diagram for the above data. Does the diagram exhibit a linear relationship? Find the predictive regression equation of blood sugar level on the distance run
Find the values of 9 and show that E(Y-P) = 0.
iv. Show the errors by drawing vertical lines between scatter points and predicted regression line.
V. Find the coefficient of determination. Comment on this finding
(b) Find Least-Squares Estimates and & in Simple Linear Regression.
Question no 5:
(n) The management of priority health club claims that its members lose an average of 10 pounds or more within the first month after joining the club. A consumer agency that wanted to check this claim took a random sample of 36 members of this health club and found that they lost an average of 9.2 pounds within the first month of membership. The population standard deviation is known to be 2.4 pounds. Use critical value approach for this test. What will your decision be if am 017 (5)
(b) According to the records of an electric company serving the Boston area, the meani electricity consumption for all houscholls during winter is 1650 kilowatt-hours per month. Assume that the monthly electricity consumptions during winter by all households in this area have a normal distribution with a mean of 1650 kilowatt-hours and a standard deviation of 320 kilowatt-hours. What percentage of the households in this area has a monthly electricity consumption of 900 to 1300 kilowatt-hours? (5)
Statistics and probability theory Sessional II in 2019
(a)Out of the total numbers of 1,807 women, who were interviewed for employment in a textile factory of Bombay, 512 were from textile areas, and the rest were from non-textile areas. Amongst the married women, who belonged for textile areas, 247 were esperienced and 73 inexperienced, while for non-textile areas, the corresponding figures were 49 and 520. The total number of inexperienced women was 1341 of whom 111 resided in the textile areas. Of the total no of women, 918 were unmarried; and of these, the no of experienced women in the textile and non-testile areas was 154 and 16 respectively, Represent the above data in a tabular form and also assign suitable title.
(b)
| Type of variable | Investment | Ordinal | Volume | Production | Speed | Cost |
| Best Measure of Central Tendancy |
Question no 2: Marks: 10
The following table gives the diastolic blood pressure reading of 250 proposers for life insurance in U.K. Calculate the Median, first Quartile and Third Quartile. Draw a suitable graph from the following figures:
| Diastolic Blood Pressure | No. of Proposers |
| 59.5-67.5 | 9 |
| 67.5-72.5 | 31 |
| 72.5-77.5 | 39 |
| 77.5-82.5 | 114 |
| 82.5-87.5 | 30 |
| 87.5-95.5 | 27 |
Final 202
Question no 1:
(a)A manufacturer of airplane parts knows from past experience that the probability is 0.80 that an order will be ready for shipment on time, and it is 0.72 that an order will be ready for shipment on time and will also be delivered on time. What is the probability that such an order will be delivered on time given that it was ready for shipment on time?
(b) Find the probability of randomly drawing two aces in succession from an ordinary deck of 52 playing cards if we sample (i) without replacement (ii) with replacement
(e) A card is chosen at random from a deck of 52 cards. It is then replaced and a second card is
Chosen. What is the probability of choosing a jack and then an eight?
Marks: 10
Question no 2:
(a)Let A be the even that a family has children of both gender and B be the event that a family has at most one boy. If a family is known to have (i) three children, then show that A and B are independent events (ii) four children, then show that A and B are dependent events.
(b) Find Least-Squares Estimates a and b in Simple Linear Regression.
Question no 3: Marks: 10
(a) Selecting a few voters from a very large population of voters and observing whether or not each of them favors a certain proposition in an election when 54% of all voters are known to be in favor of this proposition. Is this experiment a binomial experiment? Explain why.
(b) Records show that the probability is 0.00005 that a car will have a flat tire while crossing a certain bridge. Use a suitable probability distribution to find the probabilities that among 10,0000 cars crossing this bridge, (a) Exactly two will have a flat tire (b) at most two will have a flat tire.
Question no 4: Marks: 10
(a) Grand Auto Corporation produces auto batteries. The company claims that its top-of-the-line Never Die batteries are good, on average, for at least 65 months. A consumer protection agency tested 45 such batteries to check this claim It found that the mean life of these 45 batteries is 63.4 months, and the standard deviation is 3 months. Find the p-value for the test that the mean life of
Statistics and probability theory FA2020

#Statistics and probability theory project #Computer Science all subject past paper # Statistics and probability theory Problem with source code # Statistics and probability theory Course # Statistics and probability theory Project idea # Statistics and probability theory MCQ # Statistics and probability theory Interview Question #Computer Science all courses Statistics and probability theory #University Past Paper # Statistics and probability theory #Question paper #old paper

